On Contribution Of Jainology To Indian Karm Structures

Author(s): L C Jain, C K Jain
Publisher: Z_Jain_Vidya_evam_Prakrit_014026_HR.pdf

Page 7

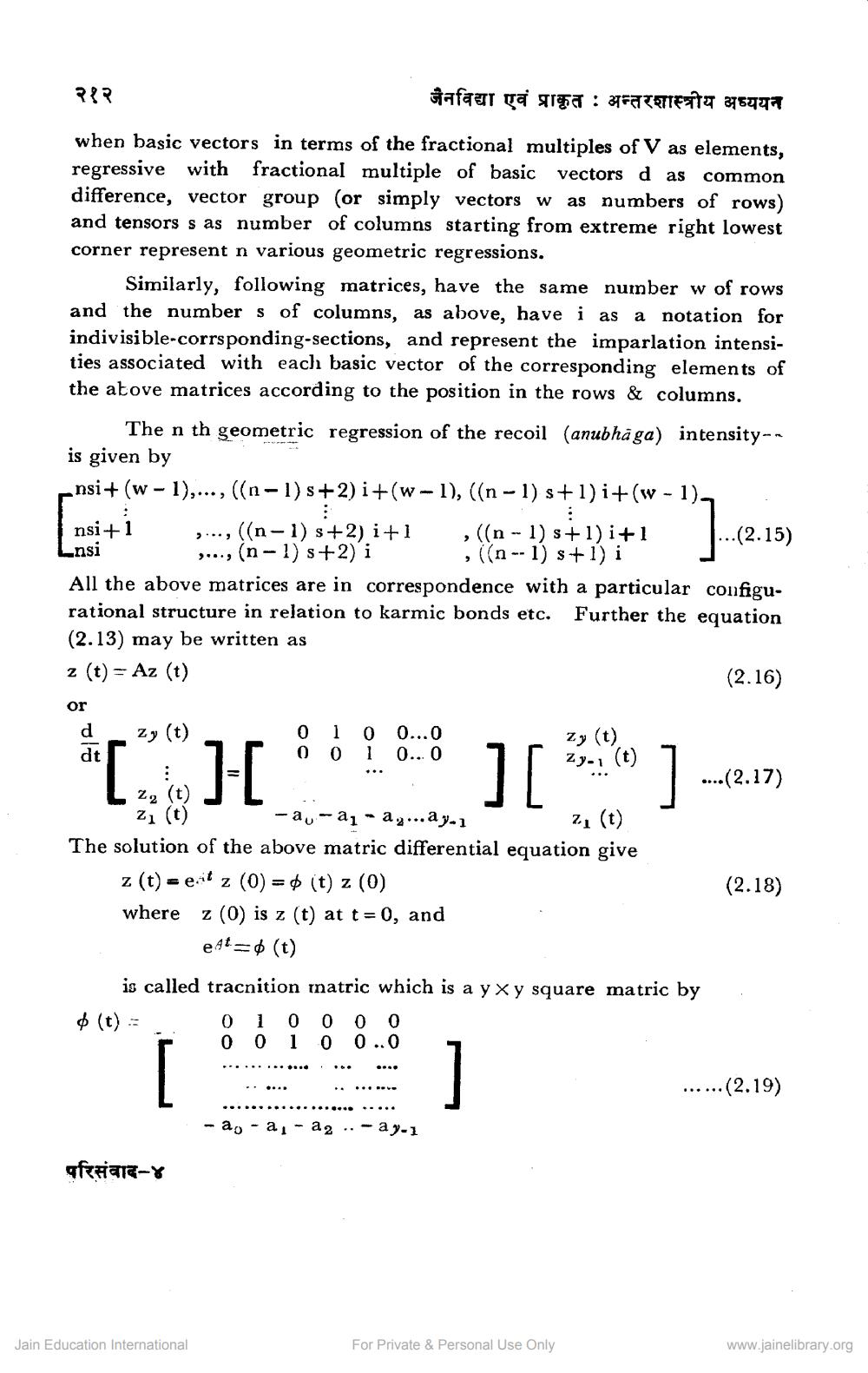
२१२
जैनविद्या एवं प्राकृत : अन्तरशास्त्रीय अध्ययन
when basic vectors in terms of the fractional multiples of V as elements, regressive with fractional multiple of basic vectors d as common difference, vector group (or simply vectors was numbers of rows) and tensors s as number of columns starting from extreme right lowest corner represent n various geometric regressions.
Similarly, following matrices, have the same number w of rows and the numbers of columns, as above, have i as a notation for indivisible-corrsponding-sections, and represent the imparlation intensities associated with each basic vector of the corresponding elements of the atove matrices according to the position in the rows & columns.
The n th geometric regression of the recoil (anubhāga) intensity-- is given by nsi+ (w – 1),..., ((n-1) s+2) i+(w – 1), ((n-1) s+1)i+(w - 1), . nsi +1 ..., ((n-1) s+2) i+1 ((n-1) s+1)i+1 ... (2.15) Lnsi ..., (n-1) s+2)'i
((n -- 1) s+1) i All the above matrices are in correspondence with a particular configurational structure in relation to karmic bonds etc. Further the equation (2.13) may be written as 2 (t) = Az (t)
(2.16)
or
d
zy (t)
dir ?, (t)
0 0
1 0
0 1
0...O 0...0
Zy (t) 2y-(t)
7
....(2.17)
L2, (t)
zi(t)
-a, -a, -a,...ay.
2 2 (t) The solution of the above matric differential equation give
z (t) = e.it z (0) = 0 (t) z (0) where z (0) is z (t) at t=0, and
eft=(t)
(2.18)
is called tracnition matric which is a y x y square matric by $(t) = .. 0 1 0 0 0 0
0 0 1 0 0.0
...... (2.19)
-a, -a, -22 ..-ay-1
परिसंवाद-४