Indian Antiquary Vol 20

SR No.: 032512
Original Sutra Author: N/A
Author: John Faithfull Fleet, Richard Carnac Temple
Publisher: Swati Publications
Publication Year: 1984
Total Pages: 486
Language: English
Classification: Book_English
File Size: 21 MB

Page 78

THE INDIAN ANTIQUARY.
[FEBRUARY, 1891.
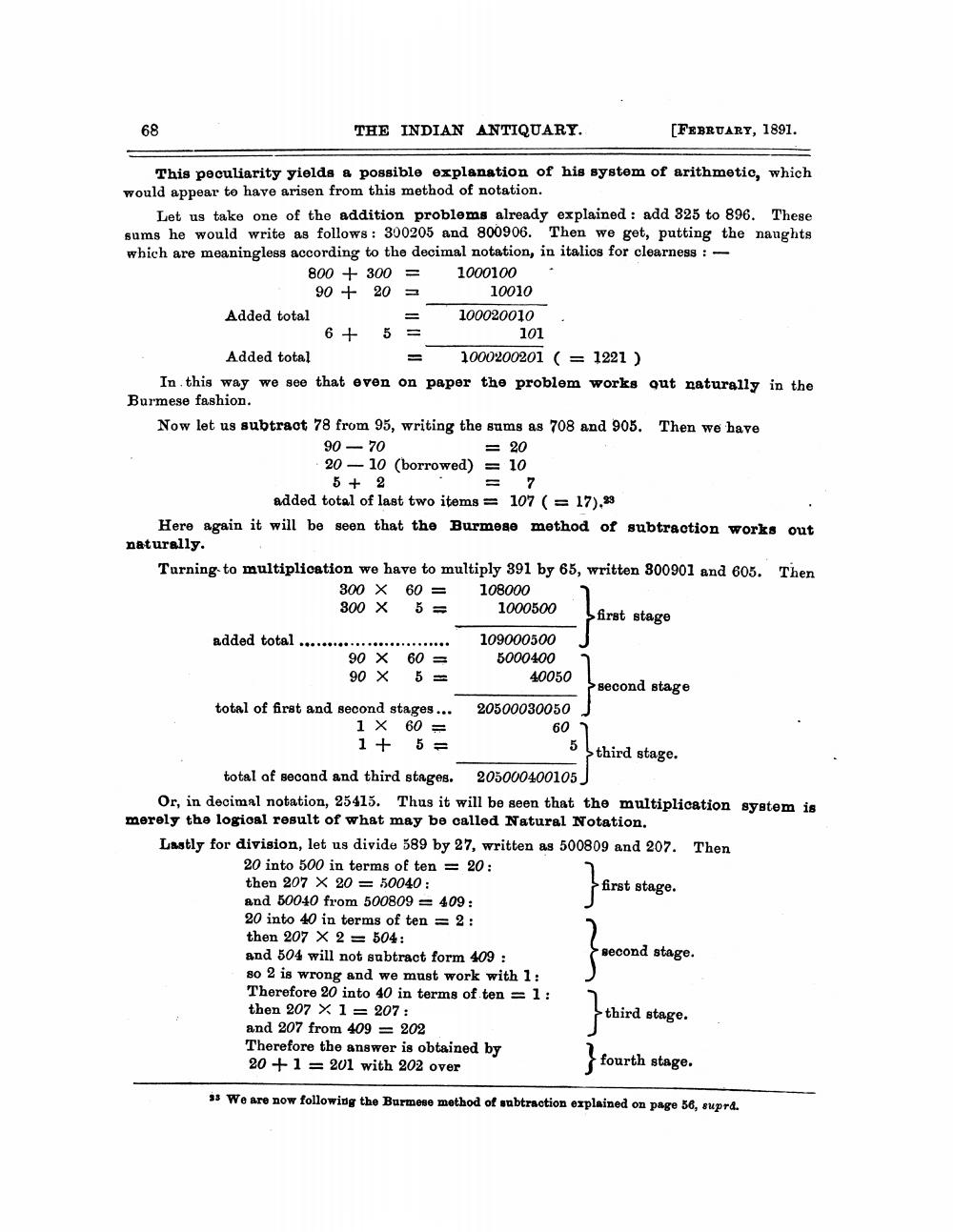
This peculiarity yields a possible explanation of his system of arithmetic, which would appear to have arisen from this method of notation.
Let us take one of the addition problems already explained : add 825 to 896. These sums he would write as follows: 300205 and 800906. Then we get, putting the naughts which are meaningless according to the decimal notation, in italios for clearness : -
800 + 300 = 1000100 90 + 20 =
10010 Added total
=
100020010 6 + 5 =
101 Added total
1000200201 ( = 1221 ) In this way we see that even on paper the problem works out naturally in the Burmese fashion. Now let us subtract 78 from 95, writing the sums as 708 and 905. Then we have 90 - 70
= 20 20 - 10 (borrowed) = 10
5 + 2
added total of last two items = 107 ( = 17).23 Here again it will be seen that the Burmese method of subtraction works out naturally. Turning to multiplication we have to multiply 391 by 65, written 800901 and 603. Then
300 X 60 = 108000 300 X 5 = 1000500
first stage added total .......
109000500 J 90 X 60 = 5000400 90 X 5 =
40050
second stage
total of first and second stages... 20500030050 J 1 X 60 =
60 1 + 5 =
$third stage. total of second and third stages. 205000400105 ) Or, in decimal notation, 25415. Thus it will be seen that the multiplication system is merely the logioal result of what may be called Natural Notation. Lastly for division, let us divide 589 by 27, written as 500809 and 207. Then
20 into 500 in terms of ten = 20 : then 207 X 20 = 50040 :
first stage. and 50040 from 500809 = 409 : 20 into 40 in terms of ten = 2: then 207 X 2 = 504: and 504 will not subtract form 409 :
second stage. 80 2 is wrong and we must work with 1: Therefore 20 into 40 in terms of ten = 1: then 207 x 1= 207 :
third stage. and 207 from 409 = 202 Therefore the answer is obtained by 20 +1 = 201 with 202 over
fourth stage.
33 We are now following the Burmese method of subtraction explained on page 56, supra.