Ganitasara Sangraha

SR No.: 020800
Original Sutra Author: N/A
Author: Mahaviracharya, M Rangacharya
Publisher: Government of Madras
Publication Year: 1912
Total Pages: 523
Language: Hindi, Sanskrit
Classification: Book_Devnagari
File Size: 22 MB

Page 409

212
GANITASĀRASANGRAHA.
figure (taken as the bējas, gives rise to the measure of the base of the (required) quadrilateral with two equal sides. The difference (between the measures of these two perpendiculars) gives the top-measure of the quadrilateral). The smaller of the diagonale (relating to the two derived figures already mentioned) gives the measure of (either of the two equal) sides. The smaller of the (two) perpendicular-sides in relation to the two derived figures under reference) gives the measure of the smaller) segment (of the base formed by the perpendicular dropped thereunto from either of the end-points of the top-side). The larger of the two) diagonals (in relation to the two derived figures of reference) gives the measure of the (required) diagonal. The area of the larger (of two derived figures of reference) is the area of the (required)
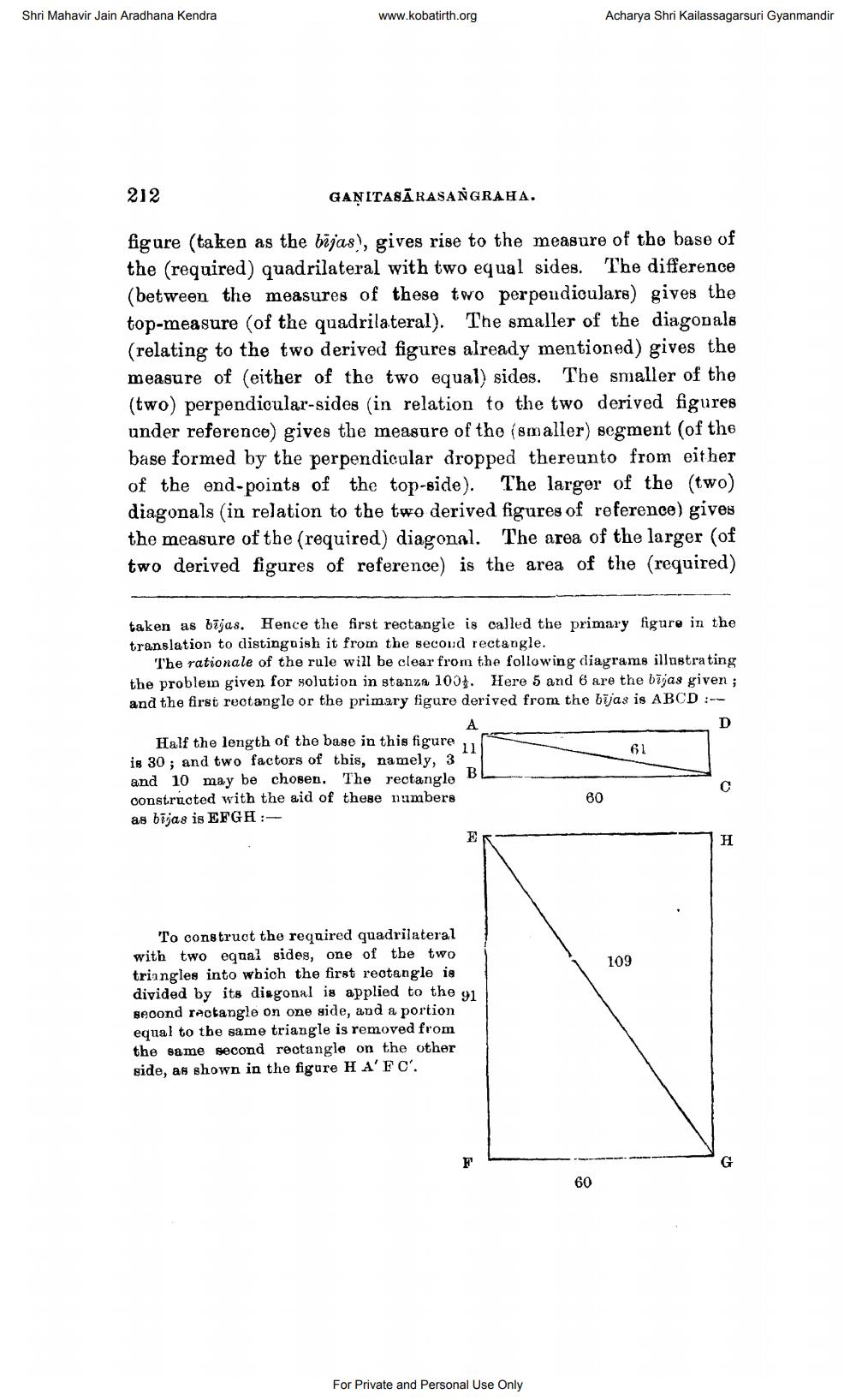
taken as bijas. Hence the first rectangle is called the primary figure in the translation to distinguish it from the secoud rectangle.
The rationale of the rule will be clear from the following diagrams illustrating the problein given for solution in stanza 100. Here 5 and 6 are the bējas given ; and the first rectangle or the primary figure derived from the bijas is ABCD :--
A Half the length of the base in this figure is 30; and two factors of this, namely, 3 and 10 may be chosen. The rectangle constructed with the aid of these numbers as bējas is EFGH:
80
109
To construct the required quadrilateral with two equal sides, one of the two triangles into which the first rectangle is divided by its diagonal is applied to the 91 second ractangle on one side, and a portion equal to the same triangle is removed from the same second rectangle on the other side, as shown in the figure HAFO'.