Ganittilakam Savrtuttikam

SR No.: 003174
Original Sutra Author: N/A
Author: Hiralal R Kapadia
Publisher: Oriental Research Institute Vadodra
Publication Year: 1937
Total Pages: 214
Language: Hindi
Classification: Book_Devnagari
File Size: 11 MB

Page 45

XLIV
INTRODUCTION
If a, b and h represent the face, the base and the altitude of an isosceles trapezium, we can have the following results:
(1) The rate of decrease of b or increase of a =574 (2) Area =} (a+b)h.
(3) At a height h' above the base, the breadth of the figure will be 6-6a l' and at a depth h” below the face, the breadth will be a+b=ah'.
It may not be amiss to mention that some geometrical figures are suggested in the following passage of Dastās'ruta. skandha (VII):
"मासियेण भिक्खुपडिमं पडिपण्णस्स अणगारस्स छव्विहा गोयरचरिया पण्णत्ता, तंजहापेडा, अद्धपेडा, गोमुत्तिया, पयंगविहिया, संबुक्कावष्टा', गंतुंपञ्चागया"
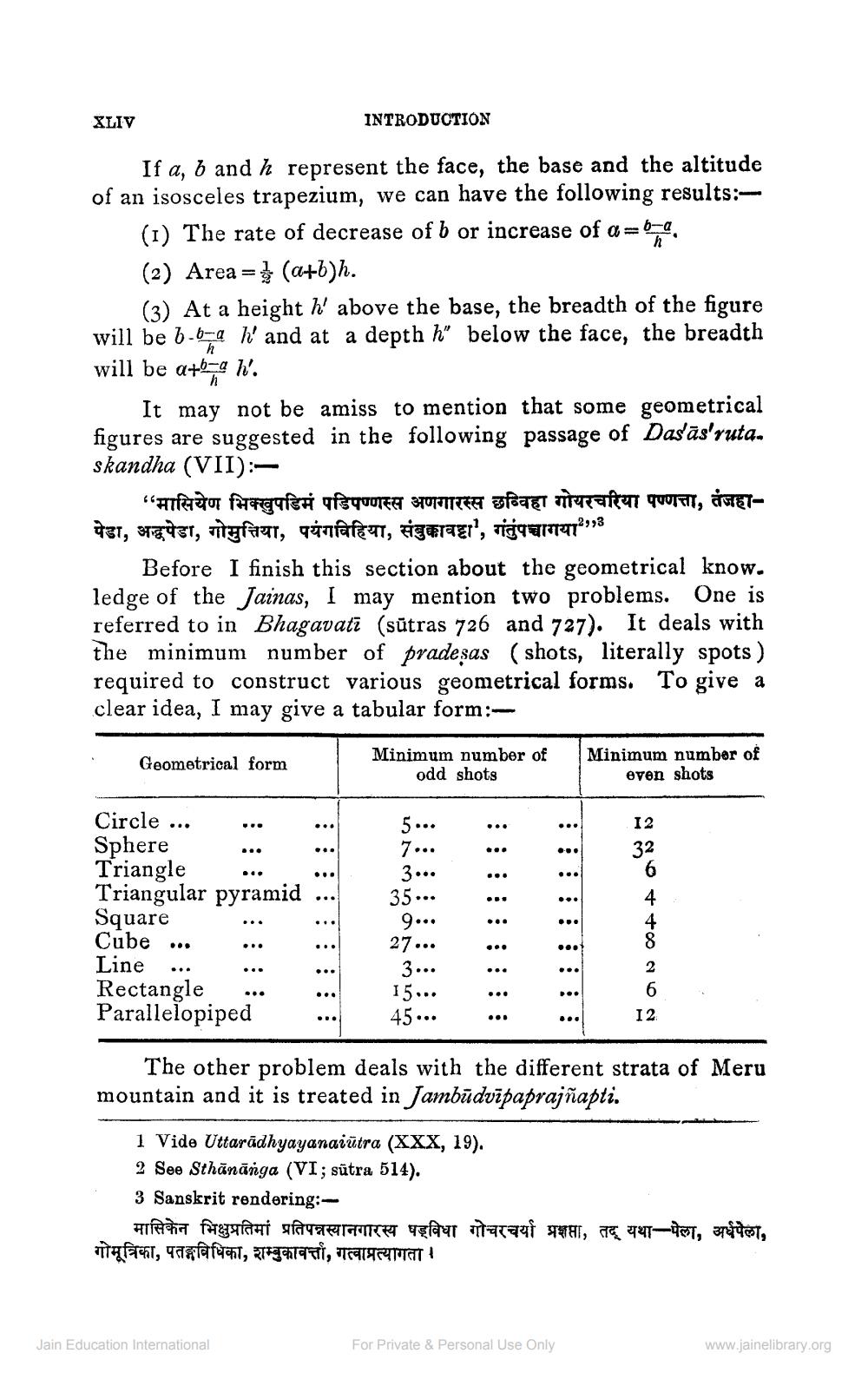
Before I finish this section about the geometrical know. ledge of the Jainas, I may mention two problems. One is referred to in Bhagavatī (sūtras 726 and 727). It deals with the minimum number of pradesas (shots, literally spots) required to construct various geometrical forms. To give a clear idea, I may give a tabular form:
Geometrical form
Minimum number of
odd shots
Minimum number of
even shots
5...
7...
3... 35...
Circle ... Sphere Triangle Triangular pyramid ... Square Cube ... Line ... Rectangle Parallelopiped ...
9...
27...
3... 15... 45...
The other problem deals with the different strata of Meru mountain and it is treated in Jambūdvīpaprajñapti.
1 Vide Uttarādhyayanaiutra (XXX, 19). 2 See Sthānanga (VI; sūtra 514). 3 Sanskrit rendering:
मासिकेन भिक्षुप्रतिमां प्रतिपन्नस्यानगारस्य षड्विधा गोचरचर्या प्रशप्ता, तद् यथा-पेला, अर्धपेला, गोमूत्रिका, पतङ्गविधिका, शम्बुकावा, गत्वाप्रत्यागता ।