Ganitasara Sangraha of Mahavira

SR No.: 011112
Original Sutra Author: N/A
Author: Rangacharya
Publisher: Rangacharya
Publication Year: 1912
Total Pages: 531
Language: English
Classification: Book_English
File Size: 24 MB

Page 420

218
GANITAS BASARGRAHA.
reference). The quotients (80 obtained) are multiplied respectively by the perpendicular-side and the base of the smaller (oblong of reference. The resulting) produots give rise to the measures of the perpendioulars (in relation to the required quadrilateral). To these (two perpendiculars), the above values of the two sides (other than the base and the top-side) are (separately) added, (the larger side being added to the larger perpendicular and the smaller side to the smaller perpendicular). The differences between these perpendiculars and sides are also obtained in the same order). The sums (above noted) are multiplied (respectively) by (these) differences. The square roots (of the products so obtained) give rise to the values of the segments (of the base in relation to the required quadrilateral). Half of the product of the diagonals (of the required quadrilateral) gives the value of its area.
The rule for arriving at an irosceles triangle with the aid of single derived oblong (of reference).
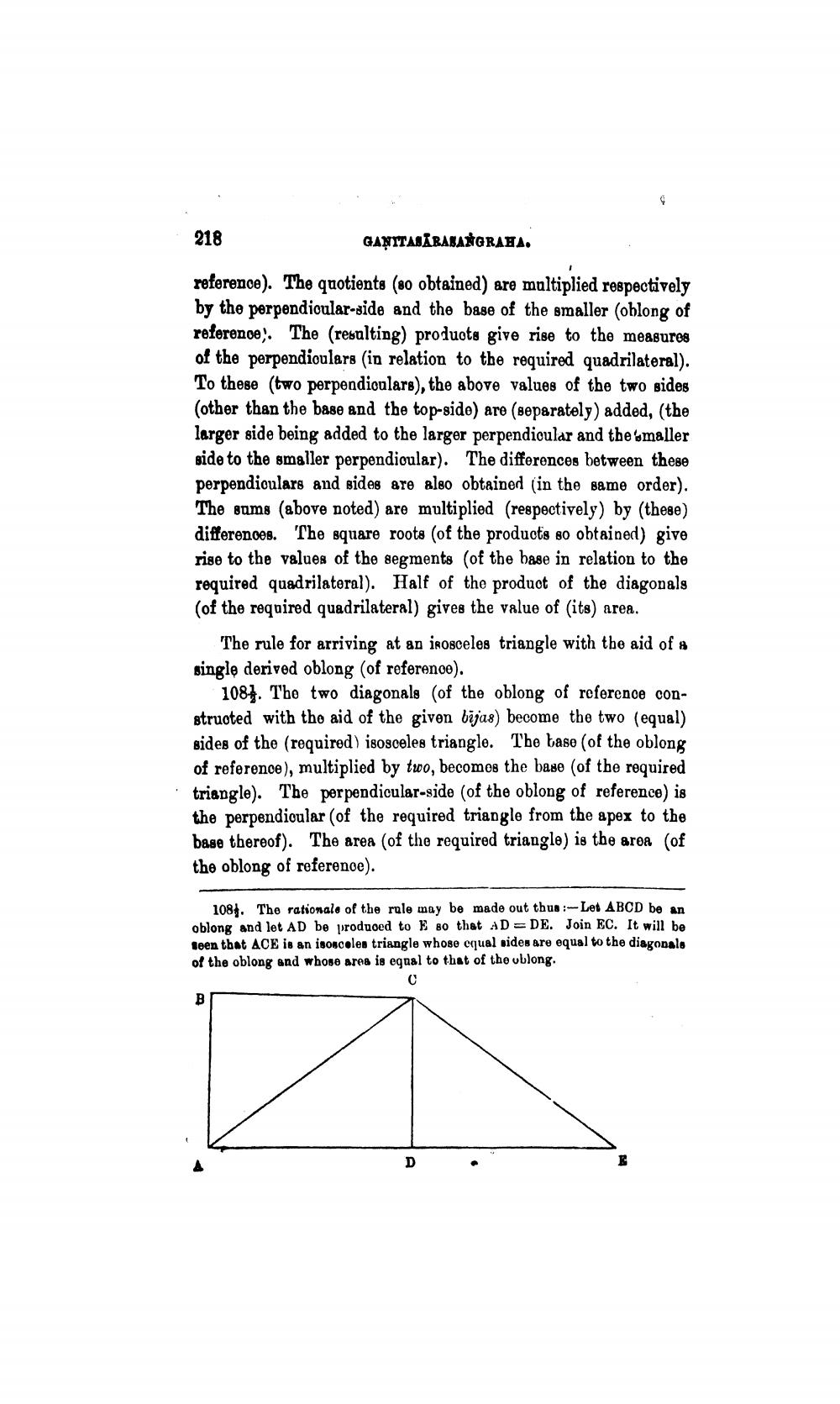
1081. The two diagonals (of the oblong of reference constructed with the aid of the given bējas) become the two (equal) sides of the required) isosceles triangle. The base (of the oblong of reference), multiplied by two, becomes the base (of the required triangle). The perpendicular-side (of the oblong of reference) is the perpendicular (of the required triangle from the apex to the base thereof). The area (of the required triangle) is the area of the oblong of referenoe).
108. The rationale of the rule may be made out thus :- Let ABCD be an oblong and let AD be produoed to E so that AD =DE. Join EC. It will be teen that ACE is an isoscoles triangle whose oqual sides are equal to the diagonale of the oblong and whose area is equal to that of the vblong.
0