Ganittilakam Savrtuttikam

SR No.: 003174
Original Sutra Author: N/A
Author: Hiralal R Kapadia
Publisher: Oriental Research Institute Vadodra
Publication Year: 1937
Total Pages: 214
Language: Hindi
Classification: Book_Devnagari
File Size: 11 MB

Page 55

LIV
From this it will be seen that this is practically the same method as given by Sridhara and Mahāvīrācārya1.
The second method is so to say as under:
Suppose we have an A.P. of which the first term is one, the common difference is also one, and the last term, the number of which the cube is required. This cube is obtained by adding together (i) the last term multiplied by 3 as well as by the preceding one (mukha), and (ii) the cube of this mukha, with one added to it. Roughly speaking, this means that if is the number of which the cube is required, then it is equal to 3(-1) r + ( v − 1 )3 + 1.3
The third method is nothing else but multiplying the given number by itself and then the result by the given number. That is to say if a is the given number, the required cube is axaxa.
The fourth method can be stated as under:
Thrice the rus'i (given number) multiplied by its two parts, when united with the cubes of each of these parts gives the required cube.
This may
be represented as below:
x3 = 3abx+a3+b3 where a and b are the two parts of the given number x.
As regards extracting cube-root, S'ripati has given only
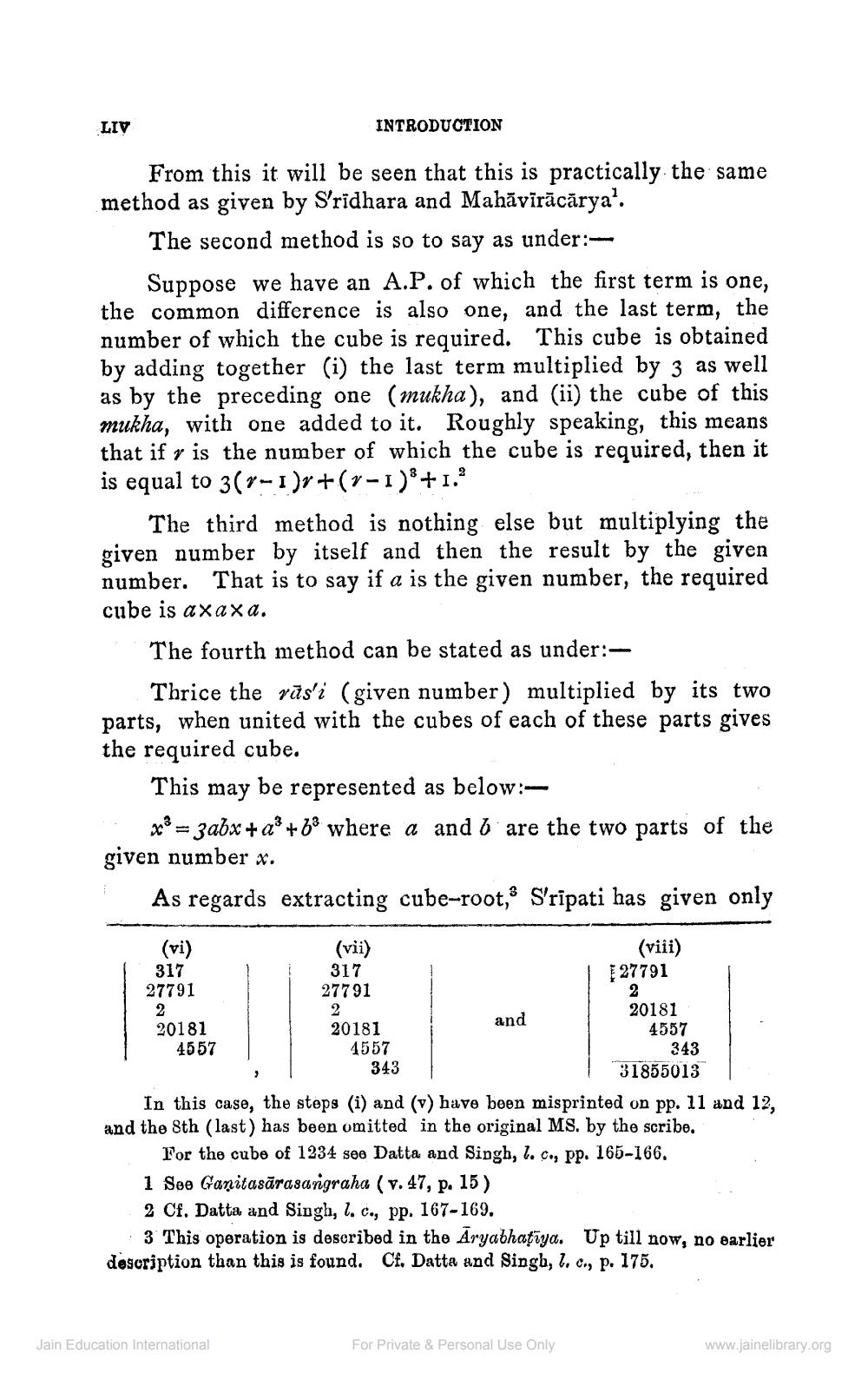
(vi)
317 27791
2 20181
INTRODUCTION
4557
(vii)
317 27791
2
20181 4557 343
and
(viii)
Į 27791
In this case, the steps (i) and (v) have been misprinted on pp. 11 and 12, and the 8th (last) has been omitted in the original MS. by the scribe.
For the cube of 1234 see Datta and Singh, l. c., pp. 165-166.
2 20181 4557 343 31855013
1 See Ganitasarasangraha (v. 47, p. 15)
2 Cf. Datta and Singh, l. c., pp. 167-169.
3 This operation is described in the Aryabhatiya. Up till now, no earlier description than this is found. Cf. Datta and Singh, l. c., p. 175.